Recommendation for individuals using a screenreader: please set your punctuation settings to "most."
Descriptive Statements:
- Demonstrate knowledge of the mole concept and its use in chemical calculations.
- Solve problems involving molar mass, percent-composition, and empirical and molecular formulas.
Sample Item:
Which of the following is equivalent to 1.42 × 10231.42 times 10 to the power of 23 atoms?
- 25.0 g Br
- 15.0 g Cu
- 19.0 g Sn
- 23.0 g Mn
Correct Response and Explanation (Show Correct ResponseHide Correct Response)
B. This question requires the examinee to demonstrate knowledge of the
mole concept. The number of moles of an element is equal to the product of the mass of
the element ÷divided by the molar mass of the element ×times Avogadro's number.
Descriptive Statements:
- Demonstrate the ability to interpret chemical notation, balance chemical equations, and recognize net ionic equations.
- Solve stoichiometric problems involving moles, mass, volume, and energy, including limiting reactant and percent yield.
Sample Item:
N2(g) + 3H2(g) → 2NH3(g)N2 left parenthesis g right parenthesis plus 3H2 left parenthesis g right parenthesis right arrow 2NH3 left parenthesis g right parenthesis
Given the equation shown above, how much NH3 is formed when 823 g of N2 are combined with 145 g of H2?
- 58.8 g
- 96.7 g
- 815 g
- 1650 g
Correct Response and Explanation (Show Correct ResponseHide Correct Response)
C. This question requires the examinee to solve stoichiometric problems
involving mass. By calculating the number of moles of each reactant and analyzing the
stoichiometric relationship between them, H2 can be identified as the limiting reactant.
From the stoichiometric relationship between H2 and NH3, the number of moles of product formed can be calculated. This mole quantity can then be converted into mass using the
molar mass of NH3.
Descriptive Statements:
- Demonstrate knowledge of different types of solutions, colloids, and suspensions.
- Solve problems involving concentrations of solutions.
- Analyze factors that affect solubility and solubility curves.
- Analyze the colligative properties of solutions.
Sample Item:
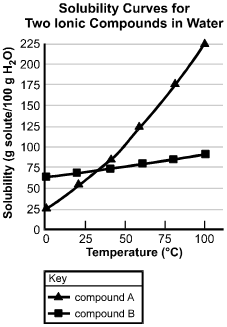
The horizontal axis is labeled Temperature in degrees centigrade, and has values marked from 0 to 100 in increments of 25. The vertical axis is labeled "Solubility (g solute/100 g H sub 2 O)", and has values marked from 0 to 225 in increments of 25. The curve for compound A passes through the following data points (values are approximate): (0, 25); (20, 55); (45, 80); (55, 125); (80, 175); and (100, 225). The curve for compound B passes through the following data points (values are approximate): (0, 65); (20, 70); (45, 75); (57, 77); (80, 80); and (100, 90).
Based on the solubility curves shown above, which of the following procedures will be
most effective in isolating the greatest amount of pure compound A from a mixture
consisting of 200 g of compound A and 15 g of compound B?
- dissolving the mixture in 100 g of water and then heating to the solution's boiling point
- dissolving the mixture in 100 g of water at 100°C100 degrees Celsius and then decreasing the temperature to 0°C0 degrees Celsius
- dissolving the mixture in 100 g of water at 75°C75 degrees Celsius, filtering the solution, and then retaining the filtrate
- dissolving the mixture in 100 g of water and then slowly increasing the temperature to 100°C100 degrees Celsius
Correct Response and Explanation (Show Correct ResponseHide Correct Response)
B. This question requires the examinee to analyze solubility curves.
Based on the solubility curves provided, both compound A and compound B will be
completely in solution when dissolved in 100 g of water at 100°C100 degrees Celsius. As the temperature
of the solution is decreased to 0°C0 degrees Celsius, 175 g of compound A will come out of the solution,
while all of compound B will remain in the solution. This is the procedure that yields
the greatest amount of pure compound A.

